CLASSIFICATIONS OF DUPIN HYPERSURFACES IN LIE SPHERE GEOMETRY*

.

Department of Mathematics and Computer Science, College of the Holy Cross,Worcester, MA 01610, USA E-mail: tcecil@holycross.edu

Abstract This is a survey of local and global classification results concerning Dupin hypersurfaces in Sn (or Rn) that have been obtained in the context of Lie sphere geometry.The emphasis is on results that relate Dupin hypersurfaces to isoparametric hypersurfaces in spheres.Along with these classification results, many important concepts from Lie sphere geometry, such as curvature spheres, Lie curvatures, and Legendre lifts of submanifolds of Sn(or Rn), are described in detail.The paper also contains several important constructions of Dupin hypersurfaces with certain special properties.

Key words Dupin hypersurfaces; isoparametric hypersurfaces; Lie sphere geometry; Lie sphere transformations; Lie curvatures

An oriented hypersurfacef:Mn-1→Sn(orRn) is said to be Dupin if:

(a) along each curvature surface, the corresponding principal curvature is constant.

The hypersurfaceMis called proper Dupin if, in addition to Condition (a), the following condition is satisfied:

(b) the numbergof distinct principal curvatures is constant onM.

An important class of proper Dupin hypersurfaces consists of the isoparametric (constant principal curvatures)hypersurfaces inSn,and those hypersurfaces inRnobtained from isoparametric hypersurfaces inSnvia stereographic projection.For example,a standard product torusS1(r)×S1(s)?S3,r2+s2=1,is an isoparametric hypersurface with two principal curvatures,and the well-known ring cyclides of Dupin[32],[9,pp148-159]inR3are obtained from standard product tori via stereographic projection.

In this paper,we will discuss various local and global classification results for proper Dupin hypersurfaces inSn(orRn) that have been obtained in the context of Lie sphere geometry.The emphasis is on results that relate Dupin hypersurfaces to isoparametric hypersurfaces in spheres.

Along with these classification results,many important concepts from Lie sphere geometry,such as curvature spheres,Lie curvatures,and Legendre lifts of submanifolds ofSn,are described in detail.The paper also contains several important constructions of Dupin hypersurfaces with certain special properties.The presentation is based primarily on the author’s book [9], and some passages are direct quotations from that book.

1 Basic Concepts of Lie Sphere Geometry

1.1 Indefinite Space Forms and Projective Space

wherex=(x1,···,xn+1) andy=(y1,···,yn+1).We will call this scalar product the Lorentz metric.A vectorxis said to be spacelike, timelike or lightlike, respectively, depending on whether (x,x) is positive, negative or zero.We will use this terminology even when we are using a metric of different signature.In Lorentz space, the set of all lightlike vectors, given by the equation,

forms a cone of revolution, called the light cone.Timelike vectors are “inside the cone” and spacelike vectors are “outside the cone.”

Ifxis a nonzero vector in Rn+11 ,letx⊥denote the orthogonal complement ofxwith respect to the Lorentz metric.Ifxis timelike, then the metric restricts to a positive definite form onx⊥, andx⊥intersects the light cone only at the origin.Ifxis spacelike, then the metric has signature (n-1,1) onx⊥, andx⊥intersects the cone in a cone of one less dimension.Ifxis lightlike,thenx⊥is tangent to the cone along the line through the origin determined byx.The bilinear form has signature (n-1,0) on thisn-dimensional plane.

Lie sphere geometry is defined in the context of real projective space Pn, so we now recall some concepts from projective geometry.We define an equivalence relation on Rn+1-{0}by settingx ?yifx=tyfor some nonzero real numbert.We denote the equivalence class determined by a vectorxby [x].Projective space Pnis the set of such equivalence classes, and it can naturally be identified with the space of all lines through the origin in Rn+1.The rectangular coordinates (x1,···,xn+1) are called homogeneous coordinates of the point[x]∈Pn, and they are only determined up to a nonzero scalar multiple.

The affine space Rncan be embedded in Pnas the complement of the hyperplane(x1=0)at infinity by the mapφ:Rn →Pngiven byφ(u)=[(1,u)].A scalar product on Rn+1, such as the Lorentz metric, determines a polar relationship between points and hyperplanes in Pn.We will also use the notationx⊥to denote the polar hyperplane of [x] in Pn, and we will call[x] the pole ofx⊥.

Ifxis a lightlike vector in Rn+1-{0}, then [x] can be represented by a vector of the form (1,u) foru ∈Rn.Then the equation (x,x) = 0 for the light cone becomesu·u= 1(Euclidean dot product), i.e., the equation for the unit sphere in Rn.Hence, the set of points in Pndetermined by lightlike vectors in Rn+1-{0}is naturally diffeomorphic to the sphereSn-1.

1.2 M¨obius Geometry of Unoriented Spheres

In this section,we study the space of all(unoriented)hyperspheres in Euclideann-dimensional space Rnand in the unit sphereSn ?Rn+1.These two spaces of spheres are closely related via stereographic projection, as we now recall.We will always assume thatn ≥2.(See [9,pp11-14] for more detail.)

We denote the Euclidean dot product of two vectorsuandvin Rnbyu·v.We first consider stereographic projectionσ: Rn →Sn-{P}, whereSnis the unit sphere in Rn+1given byy·y=1, andP=(-1,0,···,0) is the south pole ofSn.The well-known formula forσ(u) is

We next embed Rn+1into Pn+1by the embeddingφmentioned in the preceding section.Thus, we have the mapφσ:Rn →Pn+1given by

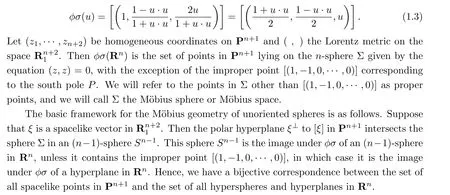
We now find specific formulas for this correspondence.Consider the sphere in Rnwith centerpand radiusr ＞0 given by the equation

We now translate this into an equation involving the Lorentz metric and the corresponding polarity relationship on Pn+1.A direct calculation shows that equation (1.4) is equivalent to the equation

whereξis the spacelike vector,

andφσ(u)is given by equation(1.3).Thus,the pointuis on the sphere given by equation(1.4)if and only ifφσ(u) lies on the polar hyperplane of [ξ].Note that the first two coordinates ofξsatisfyξ1+ξ2=1, and that (ξ,ξ)=r2.Althoughξis only determined up to a nonzero scalar multiple, we can conclude thatη1+η2is not zero for anyη ?ξ.

Conversely,given a spacelike point[z]withz1+z2nonzero,we determine the corresponding sphere in Rnas follows.Letξ=z/(z1+z2) so thatξ1+ξ2=1.Then from equation (1.6), the center of the corresponding sphere is the pointp=(ξ3,···,ξn+2), and the radius is the square root of (ξ,ξ).

We next suppose thatηis a spacelike vector withη1+η2=0.Then

In that case, the improper pointφ(P) lies on the polar hyperplane of [η], and the point [η]corresponds to a hyperplane in Rn.Again we can find an explicit correspondence as follows.

Consider the hyperplane in Rngiven by the equation

A direct calculation shows that (1.7) is equivalent to the equation

So the hyperplane (1.7) is represented in the polarity relationship by [η].Conversely, letzbe a spacelike point withz1+z2= 0.Then (z,z) =v·v, wherev= (z3,···,zn+2).Letη=z/|v|.Thenηhas the form (1.8) and [z] corresponds to the hyperplane (1.7).Thus we have explicit formulas for the bijective correspondence between the set of spacelike points in Pn+1and the set of hyperspheres and hyperplanes in Rn.

The fundamental invariant of M¨obius geometry is the angle.The study of angles in our setting is quite natural,since orthogonality between spheres and planes in Rncan be expressed in terms of the Lorentz metric.Specifically, letS1andS2denote the spheres in Rnwith respective centersp1andp2and respective radiir1andr2.By the Pythagorean Theorem, the two spheres intersect orthogonally if and only if

If these spheres correspond by equation (1.6) to the projective points [ξ1] and [ξ2], respectively, then a calculation shows that equation (1.9) is equivalent to the condition

A hyperplaneπin Rnis orthogonal to a hypersphereSprecisely whenπpasses through the center ofS.IfShas centerpand radiusr, andπis given by the equationu·N=h, then the condition for orthogonality is justp·N=h.IfScorresponds to[ξ]as in(1.6)andπcorresponds to [η] as in (1.8), then this equation for orthogonality is equivalent to (ξ,η)=0.Finally, if two planesπ1andπ2are represented by [η1] and [η2] as in (1.8), then the orthogonality conditionN1·N2=0 is equivalent to the equation (η1,η2)=0.

Note that a M¨obius transformation takes lightlike vectors to lightlike vectors, and so it induces a conformal diffeomorphism of the sphere Σ onto itself.It is well known that the group of conformal diffeomorphisms of the sphere is precisely the M¨obius group.

1.3 Lie Geometry of Oriented Hyperspheres in Rn

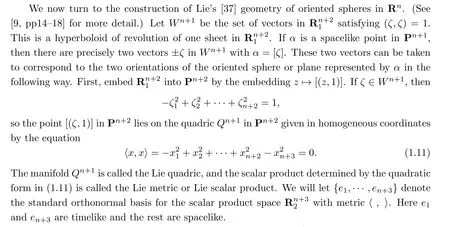
We now define a correspondence between the points onQn+1and the set of oriented hyperspheres, oriented hyperplanes and point spheres in Rn ∪{∞}.Suppose thatxis any point on the quadric with homogeneous coordinatexn+3/=0.Thenxcan be represented by a vector of the form (ζ,1), where the Lorentz scalar product (ζ,ζ)=1.Suppose first thatζ1+ζ2/=0.Then in M¨obius geometry [ζ] represents a sphere in Rn.If as in equation (1.6), we represent[ζ] by a vector of the form

then (ξ,ξ)=r2.Thusζmust be one of the vectors±ξ/r.In Pn+2, we have

We can interpret the last coordinate as a signed radius of the sphere with centerpand unsigned radiusr ＞0.In order to interpret this geometrically, we adopt the convention that a positive signed radius corresponds to the orientation of the sphere determined by the inward field of unit normals,and a negative signed radius corresponds to the orientation given by the outward field of unit normals.Hence, the two orientations of the sphere in Rnwith centerpand unsigned radiusr ＞0 are represented by the two projective points,

inQn+1.Next ifζ1+ζ2=0, then [ζ] represents a hyperplane in Rn, as in equation (1.8).Forζ= (h,-h,N), with|N| = 1, we have (ζ,ζ) = 1.Then the two projective points onQn+1induced byζand-ζare

These represent the two orientations of the plane with equationu·N=h.We make the convention that [(h,-h,N,1)] corresponds to the orientation given by the field of unit normalsN,while the orientation given by-Ncorresponds to the point [(h,-h,N,-1)]=[(-h,h,-N,1)].

Thus far we have determined a bijective correspondence between the set of pointsxinQn+1withxn+3/= 0 and the set of all oriented spheres and planes in Rn.Suppose now thatxn+3=0, i.e., consider a point [(z,0)], forz ∈Rn+21.Then〈x,x〉=(z,z)=0, and [z]∈Pn+1is simply a point of the M¨obius sphere Σ.Thus we have the following bijective correspondence between objects in Euclidean space and points on the Lie quadric:

In Lie sphere geometry,points are considered to be spheres of radius zero,or point spheres.From now on, we will use the term Lie sphere or simply “sphere” to denote an oriented sphere,an oriented plane or a point sphere in Rn ∪{∞}.We will refer to the coordinates on the right side of equation (1.14) as the Lie coordinates of the corresponding point, sphere or plane.In the case of R2and R3,respectively,these coordinates were classically called pentaspherical and hexaspherical coordinates (see [2]).

It is useful to have formulas to convert Lie coordinates back into Cartesian equations for the corresponding Euclidean object.Suppose first that [x] is a point on the Lie quadric withx1+x2/= 0.Thenx=ρy, for someρ/= 0, whereyis one of the standard forms on the right side of the table (1.14) above.From the table, we see thaty1+y2= 1, for all proper points and all spheres.Hence if we dividexbyx1+x2, the new vector will be in standard form, and we can read offthe corresponding Euclidean object from the table.In particular, ifxn+3=0,then [x] represents the pointu=(u3,···,un+2) where

Ifxn+3/= 0, then [x] represents the sphere with centerp= (p3,···,pn+2) and signed radiusrgiven by

Finally, suppose thatx1+x2=0.Ifxn+3=0, then the equation〈x,x〉=0 forcesxito be zero for 3≤i ≤n+2.Thus [x] = [(1,-1,0,···,0)], the improper point.Ifxn+3/= 0, we dividexbyxn+3to make the last coordinate 1.Then if we setN=(N3,···,Nn+2) andhaccording to

the conditions〈x,x〉=0 andx1+x2=0 forceNto have unit length.Thus [x] corresponds to the hyperplaneu·N=h, with unit normalNandhas in equation (1.17).

1.4 Oriented Hyperspheres in Sn

In some ways it is simpler to use the sphereSnrather than Rnas the base space for the study of M¨obius or Lie sphere geometry.This avoids the use of stereographic projection and the need to refer to an improper point or to distinguish between spheres and planes.Furthermore,the correspondence in the table in equation (1.14) can be reduced to a single formula (1.21)below.

As in Section 1.2, we considerSnto be the unit sphere in Rn+1, and then embed Rn+1into Pn+1by the canonical embeddingφ.Thenφ(Sn) is the M¨obius sphere Σ, given by the equation (z,z) = 0 in homogeneous coordinates.First we find the M¨obius equation for the unoriented hypersphere inSnwith centerp ∈Snand spherical radiusρ, for 0＜ρ ＜π.This hypersphere is the intersection ofSnwith the hyperplane in Rn+1given by the equation

Let [z]=φ(y)=[(1,y)].Then

Thus equation (1.18) can be rewritten as

Therefore, a pointy ∈Snis on the hyperplane determined by equation (1.18) if and only ifφ(y) lies on the polar hyperplane in Pn+1of the point

To obtain the two oriented spheres determined by equation (1.18) note that

Noting that sinρ/=0, we letζ=±ξ/sinρ.Then the point [(ζ,1)] is on the quadricQn+1, and

We can incorporate the sign of the last coordinate into the radius and thereby arrange that the oriented sphereSwith signed radiusρ/= 0,-π ＜ρ ＜π, and centerpcorresponds to a point inQn+1as follows:

The formula still makes sense if the radiusρ= 0, in which case it yields the point sphere[(1,p,0)].This one formula (1.21) plays the role of all the formulas given in equation (1.14) in the preceding section for the Euclidean case.

As in the Euclidean case, the orientation of a sphereSinSnis determined by a choice of unit normal field toSinSn.Geometrically, we take the positive radius in (1.21) to correspond to the field of unit normals which are tangent vectors to geodesics from-ptop.Each oriented sphere can be considered in two ways, with centerpand signed radiusρ,-π ＜ρ ＜π, or with center-pand the appropriate signed radiusρ±π.

Given a point [x] in the quadricQn+1, we now determine the corresponding hypersphere inSn.Multiplying by-1, if necessary, we may assume that the first homogeneous coordinatex1ofxsatisfiesx1≥0.Ifx1＞0, then we see from (1.21) that the centerpand signed radiusρ,-π/2＜ρ ＜π/2, satisfy

Ifx1=0, thenxn+3/=0, so we can divide byxn+3to obtain a point of the form (0,p,1).This corresponds to the oriented hypersphere with centerpand signed radiusπ/2, which is a great sphere inSn.

One can construct the space of oriented hyperspheres in hyperbolic spaceHnin a similar way (see [9, p18]).

1.5 Oriented Contact of Spheres

In M¨obius geometry, the principal geometric quantity is the angle.In Lie sphere geometry,the corresponding central concept is that of oriented contact of spheres.(See [9, pp19-23] for more detail and proofs of the results mentioned in this section.)

Two oriented spheresS1andS2in Rnare in oriented contact if they are tangent to each other, and they have the same orientation at the point of contact.

Ifp1andp2are the respective centers ofS1andS2, andr1andr2are their respective signed radii, then the analytic condition for oriented contact is

An oriented sphereSwith centerpand signed radiusris in oriented contact with an oriented hyperplaneπwith unit normalNand equationu·N=h, ifπis tangent toSand their orientations agree at the point of contact.Analytically, this is just the equation

Two oriented planesπ1andπ2are in oriented contact if their unit normalsN1andN2are the same.Two such planes can be thought of as two oriented spheres in oriented contact at the improper point.

A proper pointuin Rnis in oriented contact with a sphere or plane if it lies on the sphere or plane.Finally,the improper point is in oriented contact with each plane,since it lies on each plane.

Suppose thatS1andS2are two Lie spheres which are represented in the standard form given in equation (1.14) by [k1] and [k2].One can check directly that in all cases, the analytic condition for oriented contact is equivalent to the equation

It follows from the linear algebra of indefinite scalar product spaces that the Lie quadric contains projective lines but no linear subspaces of Pn+2of higher dimension(see[9,p21]).The set of oriented spheres in Rncorresponding to the points on a line onQn+1forms a so-called parabolic pencil of spheres.

The key result in establishing the relationship between the points on a line inQn+1and the corresponding parabolic pencil of spheres in Rnis the following (see [9, p22]).

Theorem 1.1 (a) The line in Pn+2determined by two points [k1] and [k2] ofQn+1lies onQn+1if and only if the the spheres corresponding to [k1] and [k2] are in oriented contact,i.e.,〈k1,k2〉=0.

(b) If the line[k1,k2]lies onQn+1,then the parabolic pencil of spheres in Rncorresponding to points on[k1,k2]is precisely the set of all spheres in oriented contact with both[k1]and[k2].

Given any timelike point [z] in Pn+2, the scalar product〈,〉 has signature (n+1,1) onz⊥.Hence,z⊥intersectsQn+1in a M¨obius space.We now show that any line on the quadric intersects such a M¨obius space at exactly one point.

Corollary 1.2 Let [z] be a timelike point in Pn+2and?a line that lies onQn+1.Then?intersectsz⊥at exactly one point.

Proof Any line in projective space intersects a hyperplane in at least one point.We simply must show that?is not contained inz⊥.This follows from the fact that〈,〉 has signature (n+1,1) onz⊥, and thereforez⊥cannot contain the 2-dimensional lightlike vector space that projects to?.□

As a consequence, we obtain the following corollary.

Corollary 1.3 Every parabolic pencil contains exactly one point sphere.Furthermore, if the point sphere is a proper point, then the pencil contains exactly one plane.

Proof The point spheres are precisely the points of intersection ofQn+1withe⊥n+3.Thus each parabolic pencil contains exactly one point sphere by Corollary 1.2.To prove the second claim, note that the oriented hyperplanes in Rncorrespond to the points in the intersection ofQn+1with (e1-e2)⊥.The line?on the quadric corresponding to the given parabolic pencil intersects this hyperplane at exactly one point unless?is contained in (e1-e2)⊥.But?is contained in(e1-e2)⊥if and only if the improper point[e1-e2]is in?⊥.This implies that the point [e1-e2] is on?.Otherwise, the 2-dimensional linear lightlike subspace of Pn+2spanned by [e1-e2] and?lies onQn+1, which is impossible.Hence, if the point sphere of the pencil is not the improper point, then the pencil contains exactly one hyperplane.□

By Corollary 1.3 and Theorem 1.1, we see that if the point sphere in a parabolic pencil is a proper pointpin Rn, then the pencil consists precisely of all spheres in oriented contact with a certain oriented planeπatp.Thus, one can identify the parabolic pencil with the point (p,N)in the unit tangent bundle to Rn, whereNis the unit normal to the oriented planeπ.If the point sphere of the pencil is the improper point, then the pencil must consist entirely of planes.Since these planes are all in oriented contact, they all have the same unit normalN.Thus the pencil can be identified with the point (∞,N) in the unit tangent bundle to Rn ∪{∞}=Sn.

It is also useful to have this correspondence between parabolic pencils and elements of the unit tangent bundleT1Snexpressed in terms of the spherical metric onSn.Suppose that?is a line on the quadric.From Corollary 1.2 and equation (1.21), we see that?intersects bothe⊥1ande⊥n+3at exactly one point.So the corresponding parabolic pencil contains exactly one point sphere and one great sphere, represented respectively by the points,

The fact that〈k1,k2〉 = 0 is equivalent to the conditionp·ξ= 0, i.e.,ξis tangent toSnatp.Hence the parabolic pencil of spheres corresponding to?can be identified with the point (p,ξ)inT1Sn.The points on the line?can be parametrized as

From equation (1.21), we see that [Kt] corresponds to the sphere inSnwith center

and signed radiust.These are precisely the spheres throughpin oriented contact with the great sphere corresponding to [k2].Their centers lie along the geodesic inSnwith initial pointpand initial velocity vectorξ.

1.6 Lie Sphere Transformations

A Lie sphere transformation is a projective transformation of Pn+2which takesQn+1to itself.In terms of the geometry of Rn, a Lie sphere transformation maps Lie spheres to Lie spheres.Furthermore, since it is projective, a Lie sphere transformation maps lines onQn+1to lines onQn+1.Thus, it preserves oriented contact of spheres in RnorSn.

Pinkall [55] proved the so-called “Fundamental Theorem of Lie sphere geometry,” which states that any line preserving diffeomorphism ofQn+1is the restriction toQn+1of a projective transformation.Thus,a transformation of the space of oriented spheres which preserves oriented contact must be a Lie sphere transformation.We will not give the proof here, but refer the reader to Pinkall’s paper [55, p431] or the book [9, p28].

Recall that a linear transformationA ∈GL(n+1) induces a projective transformationP(A) on Pndefined byP(A)[x] = [Ax].The mapPis a homomorphism ofGL(n+1) onto the groupPGL(n) of projective transformations of Pn.It is well known (see, for example,Samuel [59, p6]) that the kernel ofPis the group of all nonzero scalar multiples of the identity transformationI.

(a) Then there is a nonzero constantλsuch that〈Av,Aw〉=λ〈v,w〉 for allv,win Rnk.

(b) Furthermore, ifk/=n-k, thenλ ＞0.

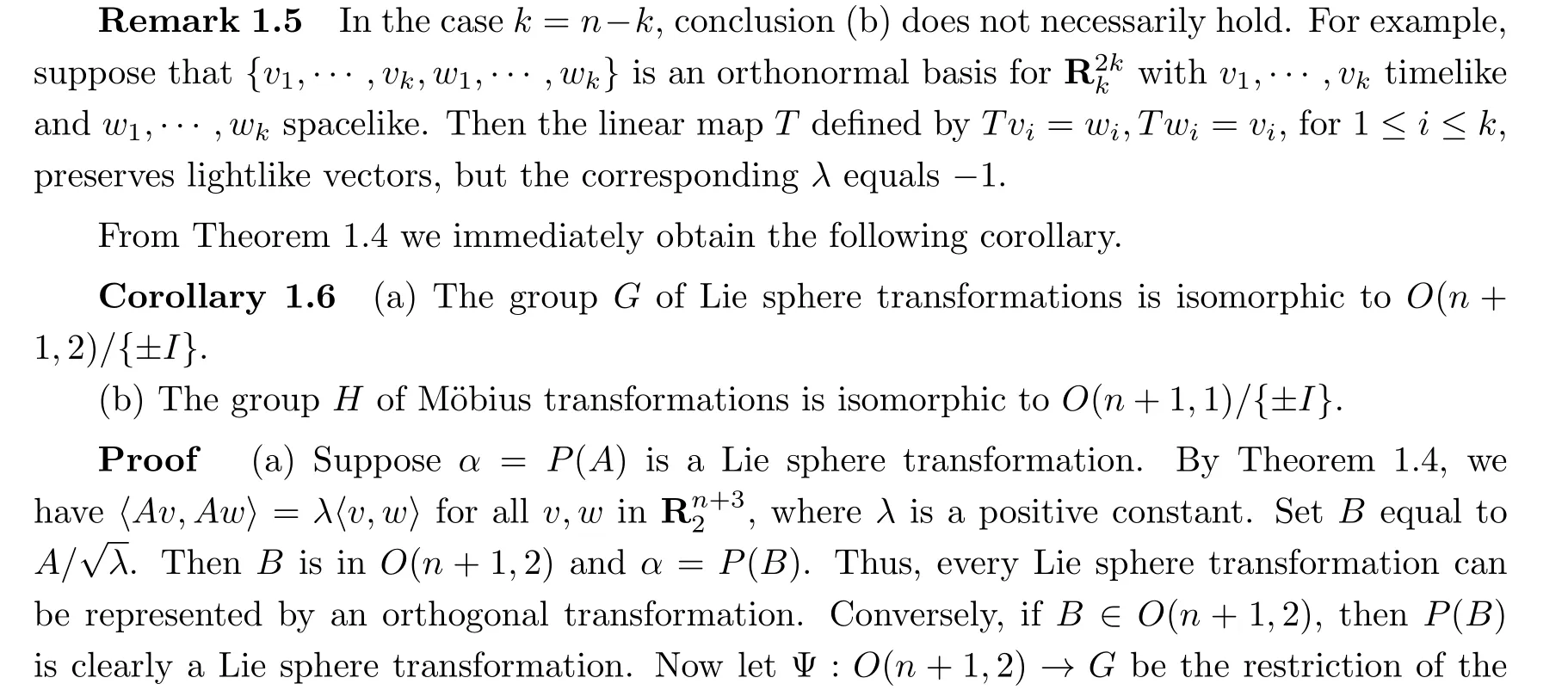
Note that whileAand-Ainduce the same M¨obius transformation, the Lie transformationP(B) is not the same as the Lie transformationP(C) induced by the matrix

where?denotes equivalence as projective transformations.Hence, the M¨obius transformationP(A) =P(-A) induces two Lie transformations,P(B) andP(C).Note thatP(B) = ΓP(C),where Γ is the change of orientation transformation represented in matrix form by

From equation (1.14), we see that Γ has the effect of changing the orientation of every oriented sphere or plane.

2 Submanifolds in Lie Sphere Geometry

In this section, we develop the framework necessary to study submanifolds of real space forms within the context of Lie sphere geometry.(See [9, pp51-64]) for more detail.) For convenience, we will deal primarily with submanifolds ofSn, and thereby avoid the complications induced by the improper point associated to Rn.This also makes it easier to treat the important class of isoparametric hypersurfaces in spheres.However, we will also consider submanifolds of Rn, when it is more convenient to do so.

2.1 Legendre Submanifolds

The manifold Λ2n-1of lines on the Lie quadricQn+1has a contact structure,i.e.,a globally defined 1-formωsuch thatω∧(dω)n-1/=0 on Λ2n-1.The conditionω=0 defines a codimension one distributionDon Λ2n-1that has integral submanifolds of dimensionn- 1, but none of higher dimension (see, for example, [9, p57]).Such an integral submanifold of maximal dimension is an immersionλ:Mn-1→Λ2n-1satisfying the conditionλ*ω= 0.It is called a Legendre submanifold [9] or a Lie geometric hypersurface [52, 55].As we shall see, these Legendre submanifolds are natural generalizations of oriented hypersurfaces inSnor Rn.

Pinkall[55](see also[9,pp59-60])showed that a smooth mapλ:Mn-1→Λ2n-1withλ=[k1,k2], for smooth mapsk1andk2fromMn-1into Rn+32, determines a Legendre submanifold if and only ifk1andk2satisfy the following conditions, which we call Pinkall’s Conditions(1)-(3).

(1)Scalar product conditions: For eachx ∈Mn-1, the vectorsk1(x)andk2(x)are linearly independent and

(2) Immersion condition: There is no nonzero tangent vectorXat any pointx ∈Mn-1such thatdk1(X) anddk2(X) are both in Span{k1(x),k2(x)}.

For eachx ∈Mn-1, [k1(x)] is the point sphere on the lineλ(x), and [k2(x)] is the great sphere onλ(x).It is easy to check that the pair{k1,k2}satisfies the conditions (1)-(3) as follows.

Condition (1) is immediate since bothfandξare maps intoSn, andξ(x) is tangent toSnatf(x) for eachxinMn-1.Condition (2) is satisfied sincedk1(X)=(0,df(X),0), for any vectorX ∈TxMn-1.Sincefis an immersion,df(X)/= 0 for a nonzero vectorX, and thusdk1(X) is not in Span{k1(x),k2(x)}.Finally, condition (3) is satisfied since for any vectorX ∈TxMn-1,〈dk1(X),k2(x)〉=df(X)·ξ(x)=0, becauseξis a field of unit normals tof.So in this case, the contact condition (3) just amounts to the fact thatξis a field of unit normals tof.The mapλis called the Legendre lift of the oriented hypersurfacefwith field of unit normalsξ.

Next, we handle the case of a submanifoldφ:V →Snof codimension greater than one.LetBn-1be the unit normal bundle of the submanifoldφ.ThenBn-1can be considered to be the submanifold ofV×Sngiven by

Geometrically,λ(x,ξ) is the line on the quadricQn+1corresponding to the parabolic pencil of spheres inSnin oriented contact at the contact element (φ(x),ξ)∈T1Sn.In this case, the point sphere map [k1] has constant rank equal to the dimension ofV.

We now return to the case of a general Legendre submanifoldλ:Mn-1→Λ2n-1.For eachx ∈Mn-1,λ(x) is a line on the quadricQn+1.This line contains exactly one point [k1(x)]corresponding to a point sphere inSnand one point [k2(x)] corresponding to a great sphere inSn.The map [k1] fromMn-1toQn+1is called the M¨obius projection or point sphere map ofλ, and likewise, the map [k2] is called the great sphere map.

The homogeneous coordinates of these points with respect to the standard basis are given by

wherefandξare both smooth maps fromMn-1toSndefined by formula (2.4).The mapfis called the spherical projection ofλ, andξis called the spherical field of unit normals.The mapsfandξdepend on the choice of orthonormal basis{e1,···,en+3}for Rn+32.For a general Legendre submanifold, the spherical projectionfis not necessarily an immersion, nor does it necessarily have constant rank.(See [9, pp59-60] for more detail.)

If the range of the point sphere map[k1]does not contain the improper point[(1,-1,0,···,0)],thenλalso determines a Euclidean projection,

According to (1.14), [Z1(x)] corresponds to the unique point sphere in the parabolic pencil determined byλ(x), and [Z2(x)] corresponds to the unique plane in this pencil.As in the spherical case, the smooth mapsFandηneed not have constant rank.(See [9, pp63-64] for more detail.)

2.2 Curvature Spheres and Dupin Hypersurfaces

To motivate the definition of a curvature sphere we consider the case of an oriented hypersurfacef:Mn-1→Snwith field of unit normalsξ:Mn-1→Sn.The shape operator offat a pointx ∈Mn-1is the symmetric linear transformationA:TxMn-1→TxMn-1defined by the equation

Often we considerfto be an embedding and suppress the mention off.Then we identify the tangent vectorXwithdf(X).

The eigenvalues ofAare the principal curvatures, and the corresponding eigenvectors are the principal vectors.We next recall the notion of a focal point of an immersion.For each real numbert, define a mapft:Mn-1→Sn, by

For eachx ∈Mn-1, the pointft(x) lies an oriented distancetalong the normal geodesic tof(Mn-1) atf(x).A pointp=ft(x) is called a focal point of multiplicitym ＞0 offatxif the nullity ofdftis equal tomatx.

The location of focal points is determined by the principal curvatures.Specifically, ifX ∈TxMn-1, then by equation (2.6) we have

Thus,dft(X) equals zero forX/=0 if and only if cottis a principal curvature offatx, andXis a corresponding principal vector.Hence,p=ft(x) is a focal point offatxof multiplicitymif and only if cottis a principal curvature of multiplicitymatx.Note that each principal curvatureκ= cott, 0＜t ＜π, produces two distinct antipodal focal points on the normal geodesic with parameter valuestandt+π.

The oriented hypersphere centered at a focal pointpand in oriented contact withf(Mn-1)atf(x) is called a curvature sphere offatx.The two antipodal focal points determined byκare the two centers of the corresponding curvature sphere.Thus, the correspondence between principal curvatures and curvature spheres is bijective.The multiplicity of the curvature sphere is by definition equal to the multiplicity of the corresponding principal curvature.

We now consider these ideas as they apply to the Legendre lift of an oriented hypersurfacefwith field of unit normalsξ.As in equation (2.1), we haveλ=[k1,k2], where

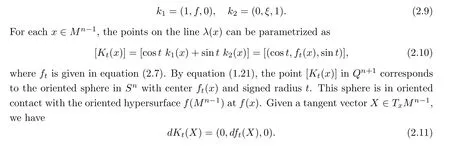
Thus,dKt(X) = (0,0,0) if and only ifdft(X) = 0, i.e.,p=ft(x) is a focal point offatx.Hence, we have shown the following.

Lemma 2.1The point [Kt(x)] inQn+1corresponds to a curvature sphere of the hypersurfacefatxif and only ifdKt(X)=(0,0,0) for some nonzero vectorX ∈TxMn-1.

This characterization of curvature spheres depends on the parametrization ofλgiven by{k1,k2}as in equation(2.9),and it has only been defined in the case where the spherical projectionfis an immersion.It is easy to show (see [9, p66]) that the following is a generalization of the definition of a curvature sphere that is valid for an arbitrary parametrization of an arbitrary Legendre submanifold.

Letλ:Mn-1→Λ2n-1be a Legendre submanifold parametrized by the pair{Z1,Z2},satisfying Pinkall’s Conditions (1)-(3) in Section 2.1.Letx ∈Mn-1andr,s ∈Rwith (r,s)/=(0,0).The sphere,

The vectorXis called a principal vector corresponding to the curvature sphere [K].

From equation (2.12), it is clear that the set of principal vectors corresponding to a given curvature sphere [K] atxis a subspace ofTxMn-1.This set is called the principal space corresponding to the curvature sphere [K].Its dimension is the multiplicity of [K].

Remark 2.2The definition of curvature sphere can be developed in the context of Lie sphere geometry without any reference to submanifolds ofSn(see Cecil-Chern[12]for details).In that case, one begins with a Legendre submanifoldλ:Mn-1→Λ2n-1and considers a curveγ(t) lying inMn-1.The set of points inQn+1lying on the set of linesλ(γ(t)) forms a ruled surface inQn+1.One then considers conditions for this ruled surface to be developable.This leads to a system of linear equations whose roots determine the curvature spheres at each point along the curve.

We next want to show that the notion of a curvature sphere is invariant under Lie sphere transformations.Letλ:Mn-1→Λ2n-1be a Legendre submanifold parametrized byλ=[Z1,Z2].Supposeβ=P(B) is the Lie sphere transformation induced by an orthogonal transformationBin the groupO(n+1,2).SinceBis orthogonal, it is easy to check that the maps,W1=BZ1,W2=BZ2, satisfy Pinkall’s Conditions (1)-(3) for a Legendre submanifold.We will denote the Legendre submanifold defined by{W1,W2}by

The Legendre submanifoldsλandβλare said to be Lie equivalent.In terms of Euclidean geometry, suppose thatVandWare two immersed submanifolds ofSn(orRn).We say thatVandWare Lie equivalent if their Legendre lifts are Lie equivalent.

Considerλandβas above,so thatλ=[Z1,Z2]andβλ=[W1,W2].Note that for a tangent vectorX ∈TxMn-1and for real numbers (r,s)/=(0,0), we have

sinceBis linear.Thus, we see that

if and only if

This immediately implies the following theorem.

Theorem 2.3Letλ:Mn-1→Λ2n-1be a Legendre submanifold andβa Lie sphere transformation.The point [K] on the lineλ(x) is a curvature sphere ofλatxif and only if the pointβ[K] is a curvature sphere of the Legendre submanifoldβλatx.Furthermore, the principal spaces corresponding to [K] andβ[K] are identical.

An important special case is when the Lie sphere transformation is a spherical parallel transformationPtdefined by,

The transformationPthas the effect of addingtto the signed radius of each sphere inSnwhile keeping the center fixed (see [9, pp48-49]).

The minus sign occurs becausePttakes a sphere with centerf-t(x) and radius-tto the point spheref-t(x).We callPtλa parallel submanifold ofλ.

Formula (2.17) shows the close correspondence between these parallel submanifolds and the parallel hypersurfacesfttof.In the case where the spherical projectionfis an immersed hypersurface, a parallel mapfthas a singularity atx ∈Mn-1if and only ifp=ft(x) is a focal point ofMn-1atx.So for eachx ∈Mn-1, there are at mostn-1 values oft ∈[0,π) for whichftfails to be an immersion.

The following theorem, due to Pinkall [55, p428] (see also [9, pp70-72]), shows that this is also true in the case where the spherical projectionfis not an immersion.This theorem is clear if the original spherical projectionfis an immersion,but it requires proof iffhas singularities.We omit the proof here, however, and refer the reader to [55, p428] or [9, pp70-72].

Theorem 2.4Letλ:Mn-1→Λ2n-1be a Legendre submanifold with spherical projectionfand spherical unit normal fieldξ.Then for eachx ∈Mn-1, the parallel map,

fails to be an immersion atxfor at mostn-1 values oft ∈[0,π).

Here [0,π) is the appropriate interval, because of the fact mentioned earlier that each principal curvature of an immersion produces two distinct antipodal focal points in the interval[0,2π).We next state some important consequences of this theorem that are obtained by passing to a parallel submanifold,if necessary,and then applying well-known results concerning immersed hypersurfaces inSn.

Corollary 2.5Letλ:Mn-1→Λ2n-1be a Legendre submanifold.Then:

(a)at each pointx ∈Mn-1,there are at mostn-1 distinct curvature spheresK1,···,Kg,

(b) the principal vectors corresponding to a curvature sphereKiform a subspaceTiof the tangent spaceTxMn-1,

(c) the tangent spaceTxMn-1=T1⊕···⊕Tg,

(d) if the dimension of a givenTiis constant on an open subsetUofMn-1, then the principal distributionTiis integrable onU,

(e) if dimTi=m ＞1 on an open subsetUofMn-1, then the curvature sphere mapKiis constant along the leaves of the principal foliationTi.

ProofIn the case where the spherical projectionfofλis an immersion, the corollary follows from known results concerning hypersurfaces inSnand the correspondence between the curvature spheres ofλand the principal curvatures off.Specifically, (a)-(c) follow from elementary linear algebra applied to the (symmetric) shape operatorAof the immersionf.As to (d) and (e), Ryan [58, p371] showed that the principal curvature functions on an immersed hypersurface are continuous.Nomizu [48] then showed that any continuous principal curvature functionκiwhich has constant multiplicity on an open subsetUinMn-1is smooth onU,as is its corresponding principal distribution (see also, Singley [63]).If the multiplicitymiofκiequals one onU, thenTiis integrable by the theory of ordinary differential equations.Ifmi ＞1, then the integrability ofTi, and the fact thatκiis constant along the leaves ofTiare consequences of Codazzi’s equation (Ryan [58], see also Cecil-Ryan [23, p24] and Reckziegel[57]).

Note that(a)-(c)are pointwise statements, while(d)-(e)hold on an open setUif they can be shown to hold in a neighborhood of each point ofU.Now letxbe an arbitrary point ofMn-1.If the spherical projectionfis not an immersion atx,then by Theorem 2.4,we can find a parallel transformationP-tsuch that the spherical projectionftof the Legendre submanifoldP-tλis an immersion atx, and hence on a neighborhood ofx.By Theorem 2.3, the corollary also holds forλin this neighborhood ofx.Sincexis an arbitrary point,the corollary is proved.

□

Letλ:Mn-1→Λ2n-1be an arbitrary Legendre submanifold.A connected submanifoldSofMn-1is called a curvature surface if at eachx ∈S, the tangent spaceTxSis equal to some principal spaceTi.For example, if dimTiis constant on an open subsetUofMn-1, then each leaf of the principal foliationTiis a curvature surface onU.Curvature surfaces are plentiful,since the results of Reckziegel [57] and Singley [63] imply that there is an open dense subset Ω ofMn-1on which the multiplicities of the curvature spheres are locally constant.On Ω, each leaf of each principal foliation is a curvature surface.

It is also possible to have a curvature surfaceSwhich is not a leaf of a principal foliation,because the multiplicity of the corresponding curvature sphere is not constant on a neighborhood ofS, as in the following example of Pinkall [55].

Example 2.6A curvature surface that is not a leaf of a principal foliation.

LetT2be a torus of revolution inR3, and embedR3intoR4=R3×R.Letηbe a field of unit normals toT2inR3.LetM3be a tube of sufficiently small radiusε ＞0 aroundT2inR4, so thatM3is a compact smooth embedded hypersurface inR4.The normal space toT2inR4at a pointx ∈T2is spanned byη(x) ande4=(0,0,0,1).The shape operatorAηofT2has two distinct principal curvatures at each point ofT2, while the shape operatorAe4ofT2is identically zero.Thus the shape operatorAζfor the normal

at a pointx ∈T2, is given by

From the formulas for the principal curvatures of a tube (see Cecil-Ryan [23, pp17-18]), one finds that at all points ofM3wherex4/=±ε, there are three distinct principal curvatures of multiplicity one, which are constant along their corresponding lines of curvature (curvature surfaces of dimension one).One of these principal curvatures isμ=-1/εresulting from the tube construction.However, on the two tori,T2×{±ε}, the principal curvatureκ= 0 has multiplicity two.These two tori are curvature surfaces for this principal curvatureκ, since the principal space corresponding toκis tangent to each torus at every point.These two tori are not leaves of a principal foliation,however,since the leaves of a foliation must all have the same dimension.The Legendre liftλof this embedding ofM3inR4has the same properties.

Part (e) of Corollary 2.5 has the following generalization, the proof of which is obtained by invoking the theorem of Ryan [58] mentioned in the proof of Corollary 2.5, with obvious minor modifications.

Corollary 2.7Suppose thatSis a curvature surface of dimensionm ＞1 in a Legendre submanifold.Then the corresponding curvature sphere is constant alongS.

As we stated at the beginning of the paper, an oriented hypersurfacef:Mn-1→Sn(orRn) is said to be Dupin if:

(a) along each curvature surface, the corresponding principal curvature is constant.

The hypersurfaceMis called proper Dupin if, in addition to Condition (a), the following condition is satisfied:

(b) the numbergof distinct principal curvatures is constant onM.

On an open subsetUon which Condition(b)holds,Condition(a)is equivalent to requiring that each curvature surface in each principal foliation be an open subset of a metric sphere inSnof dimension equal to the multiplicity of the corresponding principal curvature.Condition(a) is also equivalent to the condition that along each curvature surface, the corresponding curvature sphere map is constant.Finally, onU, Condition (a) is equivalent to requiring that for each principal curvatureκ, the image of the focal mapfκis a smooth submanifold ofSnof codimensionm+1, wheremis the multiplicity ofκ.See Cecil-Ryan [23, pp18-34] for proofs of these results.

One consequence of the results given above is that like isoparametric hypersurfaces, all proper Dupin hypersurfaces are algebraic.For simplicity, we take the ambient manifold to beRn.The theorem was formulated by Cecil, Chi and Jensen [18] as follows.

Theorem 2.8Every connected proper Dupin hypersurfacef:M →Rnembedded inRnis contained in a connected component of an irreducible algebraic subset ofRnof dimensionn-1.

Pinkall[53]sent the author a letter in 1984 that contained a sketch of a proof of this result.However,a proof was not published until 2008 by Cecil,Chi and Jensen[18],who used methods of real algebraic geometry to give a complete proof based on Pinkall’s sketch.The proof makes use of the various principal foliations whose leaves are open subsets of spheres to construct an analytic algebraic parametrization of a neighborhood off(x) for each pointx ∈M.

In contrast to the situation for isoparametric hypersurfaces, however, a connected proper Dupin hypersurface inSndoes not necessarily lie in a compact connected proper Dupin hypersurface, because the multiplicities of the principal curvatures are not necessarily constant on a compact Dupin hypersurface that contains the proper Dupin hypersurface (see Remark 2.9 below for an example).

An important class of proper Dupin hypersurfaces consists of the isoparametric hypersurfaces inSn, and those hypersurfaces inRnobtained from isoparametric hypersurfaces inSnvia stereographic projection.For example, the well-known ring cyclides of Dupin[32]inR3are obtained this way from a standard product torusS1(r)×S1(s)?S3,r2+s2=1.

The torus of revolution inR3in Example 2.6 is a cyclide of Dupin.On the torus, there areg= 2 distinct principal curvatures at each point, and each principal curvature is constant along each leaf of its corresponding principal foliation.These leaves are latitude circles for one principal curvature and longitude circles for the other principal curvature.

Remark 2.9A Dupin hypersurface that is not proper Dupin.

The tubeM3?R4over the torus in Example 2.6 is an example of a Dupin hypersurface that is not proper Dupin.At points ofM3except those on the top and bottom toriT2×{±ε},there are three distinct principal curvatures that are each constant along their corresponding principal curves(which are circles).However,onT2×{±ε},there are only two distinct principal curvatures,κ= 0 of multiplicity two, andμ=-1/εof multiplicity one.Thus,M3is not proper Dupin, since the number of distinct principal curvatures is not constant onM3.The hypersurfaceM3is Dupin, however, since along each curvature surface (includingT2×{±ε}),the corresponding principal curvature is constant.

We generalize these definitions to the context of Lie sphere geometry by defining a Legendre submanifoldλ:Mn-1→Λ2n-1to be a Dupin submanifold if:

(a) along each curvature surface, the corresponding curvature sphere is constant.

The Legendre submanifoldλis called proper Dupin if,in addition to Condition(a),the following condition is satisfied:

(b) the numbergof distinct curvature spheres is constant onM.

Of course, the Legendre lift of a Dupin hypersurface inSnorRnis Dupin in the sense defined here,but the definition here is more general,because the spherical projection of a Dupin submanifold need not be an immersion.Corollary 2.7 shows that the only curvature surfaces which must be considered in checking the Dupin property (a) are those of dimension one.

The Legendre lift of the torus of revolutionT2?R3in Example 2.6 above is a proper Dupin submanifold.On the other hand, the Legendre lift of the tubeM3overT2is Dupin,but not proper Dupin, since the number of distinct curvature spheres is not constant onM3.

The following theorem shows that both the Dupin and proper Dupin conditions are invariant under Lie sphere transformations, and many important classification results for Dupin submanifolds have been obtained in the setting of Lie sphere geometry.

Theorem 2.10Letλ:Mn-1→Λ2n-1be a Legendre submanifold andβa Lie sphere transformation.

(a) Ifλis Dupin, thenβλis Dupin.

(b) Ifλis proper Dupin, thenβλis proper Dupin.

ProofBy Theorem 2.3, a point [K] on the lineλ(x) is a curvature sphere ofλatx ∈Mif and only if the pointβ[K] is a curvature sphere ofβλatx, and the principal spaces corresponding [K] andβ[K] are identical.Since these principal spaces are the same, ifSis a curvature surface ofλcorresponding to a curvature sphere map [K], thenSis also a curvature surface ofβλcorresponding to a curvature sphere mapβ[K], and clearly [K] is constant alongSif and only ifβ[K] is constant alongS.This proves part (a) of the theorem.Part (b) also follows immediately from Theorem 2.3,since for eachx ∈M,the numbergof distinct curvature spheres ofλatxequals the number of distinct curvatures spheres ofβλatx.So if this numbergis constant onMforλ, then it is constant onMforβλ.□

2.3 Local Constructions of Dupin Hypersurfaces

Pinkall [55] introduced four constructions for obtaining a Dupin hypersurfaceWinRn+mfrom a Dupin hypersurfaceMinRn.We first describe these constructions in the casem= 1 as follows.

Begin with a Dupin hypersurfaceMn-1inRnand then considerRnas the linear subspaceRn×{0}inRn+1.The following constructions yield a Dupin hypersurfaceWninRn+1.

(1) LetWnbe the cylinderMn-1×RinRn+1.

(2)LetWnbe the hypersurface inRn+1obtained by rotatingMn-1around an axisRn-1?Rn.

(3) LetWnbe a tube of constant radius inRn+1aroundMn-1.

(4) ProjectMn-1stereographically onto a hypersurfaceV n-1?Sn ?Rn+1.LetWnbe the cone overV n-1inRn+1.

In general, these constructions introduce a new principal curvature of multiplicity one which is constant along its lines of curvature.The other principal curvatures are determined by the principal curvatures ofMn-1, and the Dupin property is preserved for these principal curvatures.These constructions can be generalized to produce a new principal curvature of multiplicitymby consideringRnas a subset ofRn×Rmrather thanRn×R.(See [9, pp127-141] for a thorough treatment of these constructions in the context of Lie sphere geometry.)

Although Pinkall defined these four constructions,his Theorem 4[55,p438]showed that the cone construction is redundant in the context of Lie sphere geometry, since it is Lie equivalent to a tube (see Remark 2.12 below).For this reason, we will restrict our attention to the three standard constructions: tubes, cylinders and surfaces of revolution.

A Dupin submanifold obtained from a lower-dimensional Dupin submanifold via one of these standard constructions is said to be reducible.More generally, a Dupin submanifold which is locally Lie equivalent to such a Dupin submanifold is called reducible.Pinkall [55,p438] proved the following Lie geometric characterization of reducibility which has been very useful in the study of Dupin hypersurfaces.

Theorem 2.11A connected proper Dupin submanifoldλ:Wn-1→Λ2n-1is reducible if and only if there exists a curvature sphere map[K]ofλthat lies in a linear subspace ofPn+2of codimension two.

ProofWe first note that the following manifolds of spheres are hyperplane sections of the Lie quadricQn+1:

a) the hyperplanes inRn,

b) the spheres with a fixed signed radiusrinRn,

c) the spheres that are orthogonal to a fixed sphere inRn.

To see this,we use the Lie coordinates given in equation(1.14).In Case a),the hyperplanes are characterized by the equationx1+x2= 0, which clearly determines a hyperplane section ofQn+1.In Case b), the spheres with signed radiusrare determined by the linear equation

In Case c),it can be assumed that the fixed sphere is a hyperplaneHthrough the origin in Rn.A sphere is orthogonal toHif and only if its center lies inH.This clearly imposes a linear condition on the vector in equation (1.14) representing the sphere.

The sets a), b), c) are each of the form

with〈w,w〉=0,-1,1 in Cases a), b), c), respectively.

We can now see that every reducible Dupin hypersurface has a family of curvature spheres that is contained in two hyperplane sections of the Lie quadric as follows.

For the cylinder construction,the tangent hyperplanes of the cylinder are curvature spheres that are orthogonal to a fixed hyperplane in Rn.Thus, that family of curvature spheres is contained in an-dimensional linear subspaceEof Pn+2such that the signature of〈,〉 on the polar subspaceE⊥ofEis (0,+).

For the surface of revolution construction,the spheres in the new family of curvature spheres all have their centers in the axis of revolution, which is a linear subspace of codimension 2 in Rn.Thus, that family of curvature spheres is contained in an-dimensional linear subspaceEof Pn+2such that the signature of〈,〉 on the polar subspaceE⊥ofEis (+,+).

For the tube construction, the spheres in the the new family of curvature spheres all have the same radius, and their centers all lie in the hyperplane of Rncontaining the manifold over which the tube is constructed.Thus, that family of curvature spheres is contained in an-dimensional linear subspaceEof Pn+2such that the signature of〈,〉 on the polar subspaceE⊥ofEis (-,+).

Conversely, suppose thatK:Wn-1→Pn+2is a family of curvature spheres that is contained in ann-dimensional linear subspaceEof Pn+2.Then〈,〉 must have signature(+,+), (0,+) or (-,+) on the polar subspaceE⊥, because otherwiseE ∩Qn+1would be empty or would consist of a single point.

If the signature ofE⊥is(+,+),then there exists a Lie sphere transformationAwhich takesEto a spaceF=A(E) such thatF ∩Qn+1consists of all spheres that have their centers in a fixed (n-2)-dimensional linear subspace Rn-2of Rn.Since one family of curvature spheres of this Dupin submanifoldAλlies inF ∩Qn+1, and the Dupin submanifoldAλis the envelope of these spheres,Aλmust be a surface of revolution with the axis Rn-2(see [9, pp142-143] for more detail on envelopes of families of spheres in this situation), and soλis reducible.

If the signature ofE⊥is (0,+), then there exists a Lie sphere transformationAwhich takesEto a spaceF=A(E) such thatF ∩Qn+1consists of hyperplanes orthogonal to a fixed hyperplane in Rn.Since one family of curvature spheres of this Dupin submanifoldAλlies inF ∩Qn+1, and the Dupin submanifoldAλis the envelope of these spheres,Aλis obtained as a result of the cylinder construction, and soλis reducible.

If the signature ofE⊥is (-,+), then there exists a Lie sphere transformationAwhich takesEto a spaceF=A(E) such thatF ∩Qn+1consists of spheres that all have the same radius and whose centers lie in a hyperplane Rn-1of Rn.Since one family of curvature spheres of this Dupin submanifoldAλlies inF ∩Qn+1, and the Dupin submanifoldAλis the envelope of these spheres,Aλis obtained as a result of the tube construction, and soλis reducible.□

Remark 2.12 When Pinkall introduced his constructions,he also listed the following cone construction.Begin with a proper Dupin submanifoldλinduced by an embedded proper Dupin hypersurfaceMn-1?Sn ?Rn+1.The new Dupin submanifoldμis the Legendre submanifold induced from the coneCnoverMn-1inRn+1with vertex at the origin.Theorem 2.11 shows that this construction is locally Lie equivalent to the tube construction as follows.As shown in Theorem 2.11, the tube construction is characterized by the fact that one curvature sphere map [K] lies in an-dimensional linear subspaceEofPn+2, whose orthogonal complement has signature(-,+).For the cone construction,the new family[K]of curvature spheres consists of hyperplanes through the origin (the point [e1+e2] in Lie sphere geometry) that are tangent to the cone along the rulings.Since the hyperplanes all pass through the improper point[e1-e2]as well as the origin[e1+e2],they correspond to points in the linear subspaceE,whose orthogonal complement is as follows:

SinceE⊥is spanned bye1ande2, it has signature (-,+).Thus, the cone construction is Lie equivalent to the tube construction.

Using these constructions, Pinkall was able to produce a proper Dupin hypersurface in Euclidean space with an arbitrary number of distinct principal curvatures, each with any given multiplicity (see Theorem 2.13 below).In general, the proper Dupin hypersurfaces constructed using Pinkall’s constructions cannot be extended to compact Dupin hypersurfaces without losing the property that the number of distinct principal curvatures is constant(see[9,pp127-141]for more detail).For now, we give a proof of Pinkall’s theorem without attempting to compactify the hypersurfaces constructed.

Theorem 2.13Given positive integersm1,···,mgwith

there exists a proper Dupin hypersurface inRnwithgdistinct principal curvatures having respective multiplicitiesm1,···,mg.

ProofThe proof is by an inductive construction, which will be clear once the first few examples are done.To begin, note that a usual torus of revolutionT2inR3is a proper Dupin hypersurface with two principal curvatures of multiplicity one.To construct a proper Dupin hypersurfaceW3inR4with three principal curvatures, each of multiplicity one, begin with an open subsetUof a torus of revolution inR3on which neither principal curvature vanishes.TakeW3to be the cylinderU×RinR3×R=R4.ThenW3has three distinct principal curvatures at each point, one of which is zero.These are clearly constant along their corresponding 1-dimensional curvature surfaces.

To get a proper Dupin hypersurface inR5with three principal curvatures having respective multiplicitiesm1=m2=1,m3=2, one simply takes

whereUis set defined above.To obtain a proper Dupin hypersurfaceZ4inR5with four principal curvatures of multiplicity one, first invert the hypersurfaceW3above in a 3-sphere inR4,chosen so that the image ofW3contains an open subsetV3on which no principal curvature vanishes.The hypersurfaceV3is proper Dupin, since the proper Dupin property is preserved by M¨obius transformations.Now takeZ4to be the cylinderV3×RinR4×R=R5.□

The proof of this theorem gives an indication of the type of problems that occur when attempting to extend these constructions to produce a compact, proper Dupin hypersurface.In particular, for the cylinder construction, the new principal curvature on the constructed hypersurfaceWnis identically zero.Thus, in order forWnto be proper Dupin, either zero is not a principal curvature at any point of the original hypersurfaceMn-1, or else zero is a principal curvature of constant multiplicity onMn-1.Otherwise, the principal curvature zero will not have constant multiplicity onWn, which implies thatWnis not proper Dupin.

2.4 Lie Curvatures of Dupin Hypersurfaces

In this section, we introduce certain natural Lie invariants of Legendre submanifolds which have been useful in the study of Dupin and isoparametric hypersurfaces.

Letλ:Mn-1→Λ2n-1be an arbitrary Legendre submanifold.As before, we can writeλ=[Y1,Y2] with

wherefandξare the spherical projection and spherical field of unit normals, respectively.At each pointx ∈Mn-1, the points on the lineλ(x) can be written in the form,

i.e., takeμas an inhomogeneous coordinate along the projective lineλ(x).Note thatk1corresponds toμ=∞.

The next two theorems give the relationship between the coordinates of the curvature spheres ofλand the principal curvatures off,in the case wherefhas constant rank.In the first theorem,we assume that the spherical projectionfis an immersion onMn-1.By Theorem 2.4,we know that this can always be achieved locally by passing to a parallel submanifold.

Theorem 2.14Letλ:Mn-1→Λ2n-1be a Legendre submanifold whose spherical projectionf:Mn-1→Snis an immersion.LetY1andY2be the point sphere and great sphere maps ofλas in equation (2.18).Then the curvature spheres ofλat a pointx ∈Mn-1are

whereκ1,···,κgare the distinct principal curvatures atxof the oriented hypersurfacefwith field of unit normalsξ.The multiplicity of the curvature sphere [Ki] equals the multiplicity of the principal curvatureκi.

ProofLetXbe a nonzero vector inTxMn-1.Then for any real numberμ,

This vector is in Span{Y1(x),Y2(x)}if and only if

i.e.,μis a principal curvature offwith corresponding principal vectorX.□

A second noteworthy case is when the point sphere mapY1is a curvature sphere of constant multiplicitymonMn-1.By Corollary 2.5, the corresponding principal distribution is a foliation, and the curvature sphere map [Y1] is constant along the leaves of this foliation.Thus the map[Y1]factors through an immersion[W1]from the space of leavesVof this foliation intoQn+1.We can write

whereφ:V →Snis an immersed submanifold of codimensionm+1.The manifoldMn-1is locally diffeomorphic to an open subset of the unit normal bundleBn-1of the submanifoldφ,andλis essentially the Legendre lift of the submanifoldφ(V),as defined in(2.2).The following theorem relates the curvature spheres ofλto the principal curvatures ofφ.Recall that the point sphere and great sphere maps forλare given as in equation (2.3) by

for (x,ξ)∈Bn-1.The proof of Theorem 2.15 is similar to the proof of Theorem 2.14 above,but it requires putting local coordinates on the unit normal bundleBn-1, and we omit it here(see [9, p74]).

Theorem 2.15 Letλ:Bn-1→Λ2n-1be the Legendre lift of the immersed submanifoldφ(V) inSnof codimensionm+1.LetY1andY2be the point sphere and great sphere maps ofλas in equation (2.20).Then the curvature spheres ofλat a point (x,ξ)∈Bn-1are

whereκ1,···,κg-1are the distinct principal curvatures of the shape operatorAξ,andκg=∞.For 1≤i ≤g-1, the multiplicity of the curvature sphere [Ki] equals the multiplicity of the principal curvatureκi, while the multiplicity of [Kg] ism.

Given these two theorems, we define a principal curvature of a Legendre submanifoldλ:Mn-1→Λ2n-1at a pointx ∈Mn-1to be a valueκin the set R∪{∞}such that[κY1(x)+Y2(x)]is a curvature sphere ofλatx, whereY1andY2are as in equation (2.18).

These principal curvatures of a Legendre submanifold are not Lie invariant,and they depend on the special parametrization forλgiven in equation (2.18).However, Miyaoka [39] pointed out that the cross-ratios of the principal curvatures are Lie invariant.

In order to formulate Miyaoka’s theorem, we need to introduce some notation.Suppose thatβis a Lie sphere transformation.The Legendre submanifoldβλhas point sphere and great sphere maps given, respectively, by

wherehandζare the spherical projection and spherical field of unit normals forβλ.Suppose that

are the distinct curvature spheres ofλat a pointx ∈Mn-1.By Theorem 2.3, the pointsβ[Ki],1≤i ≤g, are the distinct curvature spheres ofβλatx.We can write

Theseγiare the principal curvatures ofβλatx.

For four distinct numbersa,b,c,din R∪{∞}, we adopt the notation

for the cross-ratio ofa,b,c,d.We use the usual conventions involving operations with∞.For example, ifd=∞, then the expression (d-c)/(d-b) evaluates to one, and the cross-ratio[a,b;c,d] equals (a-b)/(a-c).

Miyaoka’s theorem can now be stated as follows.

Theorem 2.16 Letλ:Mn-1→Λ2n-1be a Legendre submanifold andβa Lie sphere transformation.Suppose thatκ1,···,κg,g ≥4, are the distinct principal curvatures ofλat a pointx ∈Mn-1, andγ1,···,γgare the corresponding principal curvatures ofβλatx.Then for any choice of four numbersh,i,j,kfrom the set{1,···,g}, we have

Proof The left side of equation (2.22) is the cross-ratio, in the sense of projective geometry, of the four points [Kh],[Ki],[Kj],[Kk] on the projective lineλ(x).The right side of equation (2.22) is the cross-ratio of the images of these four points underβ.The theorem now follows from the fact that the projective transformationβpreserves the cross-ratio of four points on a line.□

The cross-ratios of the principal curvatures ofλare called the Lie curvatures ofλ.A set of related invariants for the M¨obius group is obtained as follows.First, recall that a M¨obius transformation is a Lie sphere transformation that takes point spheres to point spheres.Hence the transformationβin Theorem 2.16 is a M¨obius transformation if and only ifβ[Y1] = [Z1].This leads to the following corollary of Theorem 2.16.

Corollary 2.17 Letλ:Mn-1→Λ2n-1be a Legendre submanifold andβa M¨obius transformation.Then for any three distinct principal curvaturesκh,κi,κjofλat a pointx ∈Mn-1, none of which equals∞, we have

whereγh,γiandγjare the corresponding principal curvatures ofβλat the pointx.

Proof First, note that we are using equation (2.23) to define the quantity Φ.Now sinceβis a M¨obius transformation, the point [Y1], corresponding toμ=∞, is taken byβto the pointZ1with coordinateγ=∞.Sinceβpreserves cross-ratios, we have

The corollary now follows since the cross-ratio on the left in(2.24)equals the left side of equation(2.23), and the cross-ratio on the right in (2.24) equals the right side of equation (2.23).□

A ratio Φ of the form (2.23) is called a M¨obius curvature ofλ.Lie and M¨obius curvatures have been useful in characterizing Legendre submanifolds that are Lie equivalent to Legendre lifts of isoparametric hypersurfaces in spheres, as we will see in the next section.

3 Classifications of Dupin Hypersurfaces

Many of the important classification results for Dupin hypersurfaces involve conditions under which the Dupin hypersurface is Lie equivalent to the Legendre lift of an isoparametric hypersurface in a sphere.These results will be discussed in this section.We begin with a brief summary of the classification results for isoparametric hypersurfaces in spheres.

3.1 Isoparametric Hypersurfaces in Spheres

An oriented hypersurfaceMin a real space form Rn,SnorHnis called an isoparametric hypersurface if it has constant principal curvatures (see, for example, [23, pp1-3] for other characterizations).

An isoparametric hypersurfaceMin Rncan have at most two distinct principal curvatures,andMmust be an open subset of a hyperplane,hypersphere or a spherical cylinderSk×Rn-k-1.This was first proven forn=3 by Somigliana [64] in 1919 (see also Levi-Civita [36] (1937) forn=3 and Segre [60] (1938) for arbitraryn).

Shortly after the publication of the papers of Levi-Civita and Segre,Cartan[3-6]undertook the study of isoparametric hypersurfaces in arbitrary real space-forms.Cartan showed that an isoparametric hypersurface in RnorHncan have at most two distinct principal curvatures,and he gave a local classification of isoparametric hypersurfaces in both ambient spaces (see[23, pp85-101]).

In the sphereSn,however,Cartan showed that there are many more possibilities.He found examples of isoparametric hypersurfaces inSnwith 1,2,3 or 4 distinct principal curvatures,and he classified connected isoparametric hypersurfaces withg ≤3 principal curvatures as follows.Ifg= 1, then the isoparametric hypersurfaceMis totally umbilic, and it must be an open subset of a great or small sphere.Ifg= 2, thenMmust be an open subset of a standard product of two spheres,

In the caseg=3, Cartan [4] showed that all the principal curvatures must have the same multiplicitym= 1,2,4 or 8, and the isoparametric hypersurface must be an open subset of a tube of constant radius over a standard embedding of a projective plane FP2intoS3m+1(see,for example, Cecil-Ryan [23, pp151-155]), where F is the division algebra R, C, H (quaternions),O (Cayley numbers), form=1,2,4,8, respectively.Thus, up to congruence, there is only one such family for each value ofm.

Cartan’s theory was further developed by Nomizu[49,50],Takagi and Takahashi[67],Ozeki and Takeuchi [51], and most extensively by M¨unzner [44, 45] (see also the English translations[44, 45]), who showed that the numbergof distinct principal curvatures of an isoparametric hypersurface must be 1,2,3,4 or 6.For more detail on the theory of isoparametric hypersurfaces,see Chapter 3 of Cecil-Ryan [23] or Chapter 3 of [22].

In the case of an isoparametric hypersurface with four principal curvatures,M¨unzner proved that the principal curvatures can have at most two distinct multiplicitiesm1,m2.Next Ferus,Karcher and M¨unzner[33](see also the English translation[33])used representations of Clifford algebras to construct for any positive integerm1an infinite series of isoparametric hypersurfaces with four principal curvatures having respective multiplicities(m1,m2),wherem2is nondecreasing and unbounded in each series.(These examples are also described in[9, pp95-112]and[23,pp162-180].)

As later work by several researchers would show(see below),this class of FKM-type isoparametric hypersurfaces contains all isoparametric hypersurfaces with four principal curvatures with the exception of two homogeneous examples, having multiplicities (2,2) and (4,5).This construction of Ferus,Karcher and M¨unzner was a generalization of an earlier construction due to Ozeki and Takeuchi [51].

Stolz [65] next proved that the multiplicities (m1,m2) of the principal curvatures of an isoparametric hypersurface with four principal curvatures must be the same as those of the hypersurfaces of FKM-type or the two homogeneous exceptions.Cecil, Chi and Jensen [15]then showed that if the multiplicities of an isoparametric hypersurface with four principal curvatures satisfym2≥2m1-1, then the hypersurface is of FKM-type.(A different proof of this result, using isoparametric triple systems, was given later by Immervoll [35].)

Taken together with known results of Takagi [66] form1=1, and Ozeki and Takeuchi [51]form1=2,this result of Cecil,Chi and Jensen handled all possible pairs of multiplicities except for four cases, the homogeneous pair (4,5), and the FKM pairs (3,4),(6,9) and (7,8).In a series of recent papers, Chi [24-29] completed the classification of isoparametric hypersurfaces with four principal curvatures.Specifically, Chi showed that in the cases (3,4), (6,9) and(7,8), the isoparametric hypersurface must be of FKM-type, and in the case (4,5), it must be homogeneous.

In the case of an isoparametric hypersurface with six principal curvatures,M¨unzner showed that all of the principal curvatures must have the same multiplicitym, and Abresch [1] showed thatmmust equal 1 or 2.By the classification of homogeneous isoparametric hypersurfaces due to Takagi and Takahashi [67], there is only one homogeneous family in each case up to congruence.These homogeneous examples have been shown to be the only isoparametric hypersurfaces in the caseg=6 by Dorfmeister and Neher [31] in the case of multiplicitym=1,and by Miyaoka [41, 42] in the casem= 2 (see also the papers of Siffert [61, 62]).See the surveys of Thorbergsson [69], Cecil [10], and Chi [30] for more information on isoparametric hypersurfaces in spheres.

3.2 Compact Proper Dupin Hypersurfaces

There is a close relationship between the theory of isoparametric hypersurfaces and the theory of compact proper Dupin hypersurfaces embedded inSn(or Rn).Thorbergsson [68]showed that the restrictiong=1,2,3,4 or 6 on the number of distinct principal curvatures of an isoparametric hypersurface also holds for a connected, compact proper Dupin hypersurfaceMembedded inSn ?Rn+1.He first showed thatMmust be taut, i.e., every nondegenerate distance functionLp(x) =d(p,x)2, whered(p,x) is the distance fromptoxinSn, has the minimum number of critical points required by the Morse inequalities onM.Using tautness,he then showed thatMdividesSninto two ball bundles over the first focal submanifolds on either side ofM.This topological situation is what is required for M¨unzner’s proof of the restriction ong.(See also [23, 65-74].)

M¨unzner’s argument also produces certain restrictions on the cohomology and the homotopy groups of isoparametric hypersurfaces.These restrictions necessarily apply to compact proper Dupin hypersurfaces by Thorbergsson’s result.Grove and Halperin [34] later found more topological similarities between these two classes of hypersurfaces.Furthermore,the results of Stolz[65] and Grove-Halperin [34] on the possible multiplicities of the principal curvatures actually only require the assumption thatMis a compact proper Dupin hypersurface, and not that the hypersurface is isoparametric.

The close relationship between these two classes of hypersurfaces led to the widely held conjecture that every compact proper Dupin hypersurfaceM ?Snis Lie equivalent to an isoparametric hypersurface (see [22, p184]).We now describe the results that have been obtained concerning this conjecture.(See [11] for a full account.)

The conjecture is obviously true forg= 1, in which case the compact proper Dupin hypersurfaceM ?Snmust be a hypersphere inSn, and soMitself is isoparametric.In 1978,Cecil and Ryan [21] showed that ifg= 2, thenMmust be an (n-1)-dimensional cyclide of Dupin, and it is therefore M¨obius equivalent to an isoparametric hypersurface inSn.Then in 1984, Miyaoka [38] showed that the conjecture is true forg= 3, that is,Mmust be Lie equivalent an isoparametric hypersurface, althoughMis not necessarily M¨obius equivalent to an isoparametric hypersurface.Thus, asgincreases, the group needed to obtain equivalence with an isoparametric hypersurface gets progressively larger.

Forg= 4, the conjecture remained open for several years until finally in 1988, counterexamples to the conjecture were discovered independently by Pinkall and Thorbergsson [56] and by Miyaoka and Ozawa [43].The two types of counterexamples are different, and the method of Miyaoka and Ozawa also yields counterexamples to the conjecture withg= 6 principal curvatures.

For both the counterexamples of Miyaoka-Ozawa and those of Pinkall-Thorbergsson, the hypersurfaces constructed do not have constant Lie curvatures, and so they cannot be Lie equivalent to an isoparametric hypersurface.(See also [9, pp112-123] or [23, pp308-322] for a description of these counterexamples to the conjecture.)

This led to the following revised version of the conjecture due to Cecil,Chi and Jensen[16],[17] that includes the assumption of constant Lie curvatures.

Conjecture 3.1 Every compact, connected proper Dupin hypersurface inSnwithg=4 org=6 principal curvatures and constant Lie curvatures is Lie equivalent to an isoparametric hypersurface.

Research on this revised conjecture has been an important part of the field, since the publication of the counterexamples to the original conjecture.A useful result in this area is the following local Lie geometric characterization of Legendre submanifolds that are Lie equivalent to the Legendre lift of an isoparametric hypersurface inSn(see [7] or [9, p77]).

Recall that a line in Pn+2is called timelike if it contains only timelike points.This means that an orthonormal basis for the 2-plane in Rn+32determined by the timelike line in Pn+2consists of two timelike vectors.An example is the line [e1,en+3].

Theorem 3.2 Letλ:Mn-1→Λ2n-1be a Legendre submanifold withgdistinct curvature spheres [K1],···,[Kg] at each point.Thenλis Lie equivalent to the Legendre lift of an isoparametric hypersurface inSnif and only if there existgpoints [P1],···,[Pg] on a timelike line in Pn+2such that

Proof Ifλis the Legendre lift of an isoparametric hypersurface inSn, then all the spheres in each family [Ki] have the same radiusρi, where 0＜ρi ＜π.By formula (1.21), this is equivalent to the condition〈Ki,Pi〉=0, where the

aregpoints on the timelike line[e1,en+3].Since a Lie sphere transformation preserves curvature spheres, timelike lines and the polarity relationship, the same is true for any image ofλunder a Lie sphere transformation.

Conversely, suppose that there existgpoints [P1],···,[Pg] on a timelike line?such that

Letβbe a Lie sphere transformation that maps?to the line [e1,en+3].Then the curvature spheresβ[Ki] ofβλare respectively orthogonal to the points [Qi]=β[Pi] on the line [e1,en+3].This means that for each value ofi,the spheres corresponding toβ[Ki]have constant radius onMn-1.By applying a parallel transformation, if necessary, we can arrange that none of these curvature spheres has radius zero.Thenβλis the Legendre lift of an isoparametric hypersurface inSn.□

Remark 3.3 In the case whereλis Lie equivalent to the Legendre lift of an isoparametric hypersurface inSn, one can say more about the position of the points [P1],···,[Pg] on the timelike line?.M¨unzner [44, 45] showed that the radiiρiof the curvature spheres of an isoparametric hypersurface must be of the form

for someρ1∈(0,π/g).Hence, after Lie sphere transformation, the [Pi] must have the form(3.1) forρias in equation (3.2).

Since the principal curvatures are constant on an isoparametric hypersurface, the Lie curvatures are also constant.By M¨unzner’s work, the distinct principal curvaturesκi,1≤i ≤g,of an isoparametric hypersurface must have the form

forρias in equation (3.2).Thus the Lie curvatures of an isoparametric hypersurface can be determined.We can order the principal curvatures so that

In the caseg=4, this leads to a unique Lie curvature Ψ defined by

The ordering of the principal curvatures implies that Ψ must satisfy 0＜Ψ＜1.Using equations(3.3)and(3.5),one can compute that Ψ=1/2 on any isoparametric hypersurface,i.e.,the four curvature spheres form a harmonic set in the sense of projective geometry (see, for example,[59, p59]).

There is,however,a simpler way to compute Ψ.One applies Theorem 2.15 to the Legendre lift of one of the focal submanifolds of the isoparametric hypersurface.By the work of M¨unzner,each isoparametric hypersurfaceMn-1embedded inSnhas two distinct focal submanifolds,each of codimension greater than one.The hypersurfaceMn-1is a tube of constant radius over each of these focal submanifolds.Therefore, the Legendre lift ofMn-1is obtained from the Legendre lift of either focal submanifold by parallel transformation.Thus, the Legendre lift ofMn-1has the same Lie curvature as the Legendre lift of either focal submanifold.Letφ:V →Snbe one of the focal submanifolds.By the same calculation that yields equation(3.2),M¨unzner showed that ifξis any unit normal toφ(V) at any point, then the shape operatorAξhas three distinct principal curvatures,

By Theorem 2.15, the Legendre lift ofφhas a fourth principal curvatureκ4=∞.Thus, the Lie curvature of this Legendre submanifold is

In the caseg= 4, one can ask what is the strength of the assumption Ψ = 1/2 onMn-1.Since Ψ is only one function of the principal curvatures, one would not expect this assumption to classify Legendre submanifolds up to Lie equivalence.However, if one makes additional assumptions, e.g., the Dupin condition, then results can be obtained.

Miyaoka[39]proved that the assumption that Ψ is constant on a compact connected proper Dupin hypersurfaceMn-1inSnwith four principal curvatures, together with an additional assumption regarding intersections of leaves of the various principal foliations, implies thatMn-1is Lie equivalent to an isoparametric hypersurface.This proved that Conjecture 3.1 is true under Miyaoka’s additional assumptions.However, no one has proved that Miyaoka’s additional assumptions always hold.In the same paper, Miyaoka also proved that if the Lie curvature of a compact proper Dupin hypersurface withg=4 is constant, then it has the value 1/2.

As mentioned above, Thorbergsson [68] showed that for a compact proper Dupin hypersurface inSnwith four principal curvatures, the multiplicities of the principal curvatures must satisfym1=m3,m2=m4, when the principal curvatures are ordered as in equation (3.4), the same restriction as for an isoparametric hypersurface (see, for example, [23, p108]).

Cecil, Chi and Jensen [16] then used a different approach than Miyaoka [39] to try to prove Conjecture 3.1.First, they proved that the Legendre lift of a connected, compact proper Dupin hypersurface withg ＞2 principal curvatures is irreducible (in the sense of Pinkall [55],see Section 2.3).Then they worked locally using the method of moving frames and assuming irreducibility to prove the following theorem.

Theorem 3.4LetMbe an irreducible connected proper Dupin hypersurface inSnwith four principal curvatures having multiplicitiesm1=m3≥1,m2=m4= 1, and constant Lie curvature Ψ = 1/2.ThenMis Lie equivalent to an open subset of an isoparametric hypersurface.

Theorem 3.4 implies the following result in the compact case due to Cecil, Chi and Jensen[16]:

Theorem 3.5LetMbe a compact, connected proper Dupin hypersurface inSnwith four principal curvatures having multiplicitiesm1=m3≥1,m2=m4= 1, and constant Lie curvature.ThenMis Lie equivalent to an isoparametric hypersurface.

Theorem 3.4 implies Theorem 3.5 for the following reasons.As noted above,Cecil,Chi and Jensen [16] proved that the Legendre lift of a connected, compact proper Dupin hypersurface withg ＞2 principal curvatures is irreducible.Next Thorbergsson’s [68] result proves that the multiplicities of a compact,connected proper Dupin hypersurface with four principal curvatures satisfy the conditionsm1=m3andm2=m4, when the principal curvatures are appropriately ordered.Finally, Miyaoka [39] proved that if the Lie curvature of a compact proper Dupin hypersurface withg= 4 is constant, then it has the value 1/2.Thus, the hypotheses of Theorem 3.5 imply that the hypotheses of Theorem 3.4 are satisfied.

This means that the full Conjecture 3.1 forg=4 would be proven, if the assumption that the value ofm2=m4is equal to one could be eliminated from Theorem 3.4, and thus from Theorem 3.5.

The proof of Theorem 3.4 involves some complicated calculations, which would become even more elaborate if the value ofm2=m4were allowed to be greater than one.Even so, this approach to proving Conjecture 3.1 could possibly be successful with some additional insight regarding the structure of the calculations involved.The criteria for reducibility (Theorem 2.11) and the criteria for Lie equivalence to an isoparametric hypersurface (Theorem 3.2) are both important in the proof of Theorem 3.4.

In the caseg=6, we do not know of any results beyond those of Miyaoka[40], who showed that if the Dupin hypersurface satisfies some additional assumptions on the intersections of the leaves of the various principal foliations, then Conjecture 3.1 is true forg=6.However, no one has proved that Miyaoka’s additional assumptions always hold.

A local approach assuming irreducibility, similar to that used by Cecil, Chi and Jensen for theg=4 case, might possibly work for the caseg=6, but the calculations involved would be very complicated, unless some new algebraic insight is found to simplify the situation.

3.3 Examples with Constant Lie Curvature That Are not Lie Equivalent to an Isoparametric Hypersurface

The following example[7](see also[9,pp80-82])is a noncompact proper Dupin hypersurface withg= 4 distinct principal curvatures of multiplicity one, and constant Lie curvature Ψ =1/2, which is not Lie equivalent to an open subset of an isoparametric hypersurface with four principal curvatures inSn.

This example is reducible in the sense of Pinkall (see Section 2.3), and it cannot be made compact without destroying the property that the numbergof distinct curvatures spheres equals four at each point.This example shows that some additional hypotheses (either compactness or irreducibility)besides constant Lie curvature Ψ=1/2 are needed to conclude that a proper Dupin hypersurface withg=4 principal curvatures is Lie equivalent to an isoparametric hypersurface.

Example 3.6 Letφ:V →Sn-mbe an embedded Dupin hypersurface inSn-mwith field of unit normalsξinSn-m, such thatφhas three distinct principal curvatures,

at each point ofV.EmbedSn-mas a totally geodesic submanifold ofSn, and letBn-1be the unit normal bundle of the submanifoldφ(V) inSn.Letλ:Bn-1→Λ2n-1be the Legendre lift of the submanifoldφ(V) inSn.Any unit normalηtoφ(V) at a pointx ∈Vcan be written in the form

whereζis a unit normal toSn-minSn.Since the shape operatorAζ=0, we have

Thus the principal curvatures ofAηare

Ifη·ξ=cosθ/=0, thenAηhas three distinct principal curvatures.However, ifη·ξ=0, thenAη=0.

LetUbe the open subset ofBn-1on which cosθ ＞0, and letαdenote the restriction ofλtoU.By Theorem 2.15,αhas four distinct curvature spheres at each point ofU.Sinceφ(V)is Dupin inSn-m, it is easy to show thatαis Dupin (see the tube construction [9, pp127-133]for the details).Furthermore, sinceκ4=∞, the Lie curvature Ψ ofαat a point (x,η) ofUequals the M¨obius curvature Φ(κ1,κ2,κ3).Using equation (3.6), we compute

Now consider the special case whereφ(V)is a minimal isoparametric hypersurface inSn-mwith three distinct principal curvatures.By M¨unzner’s formula(3.2),these principal curvatures must have the values,

On the open subsetUofBn-1described above, the Lie curvature ofαhas the constant value 1/2 by equation(3.7).To construct an immersed proper Dupin hypersurface with four principal curvatures and constant Lie curvature Ψ=1/2 inSn, we simply take the open subsetP-tα(U)of the tube of radiustaroundφ(V) inSnfor an appropriate value oft.

We can see that this example is not Lie equivalent to an open subset of an isoparametric hypersurface inSnwith four distinct principal curvatures as follows.Note that the example that we have constructed is reducible, because it is obtained by the tube construction from the hypersurfaceφ(V) inSn-m ?Sn.On the other hand, as we will show in the next paragraph, any open subset of an isoparametric hypersurface with four principal curvatures inSnis irreducible, so it cannot be Lie equivalent to our example.

The irreducibility of an open subset of an isoparametric hypersurface with four principal curvatures inSnfollows from the results of Cecil, Chi and Jensen [16], who proved that a compact proper Dupin hypersurface withg ＞2 principal curvatures is irreducible.Furthermore,they proved that an irreducible proper Dupin hypersurface cannot contain a reducible open subset.This follows from the algebraicity of proper Dupin hypersurfaces, as stated in Theorem 2.8 (see also [9, pp145-147]).Thus, a compact isoparametric hypersurface with four principal curvatures inSnis irreducible, and it cannot contain a reducible open subset.

Note that the point sphere map[Y1]of the Legendre submanifoldλ:Bn-1→Λ2n-1defined above is a curvature sphere of multiplicitymwhich lies in the linear subspace of codimensionm+1 in Pn+2orthogonal to the space spanned byen+3and by those vectorsζnormal toSn-minSn.This geometric fact implies that for such a vectorζ, there are only two distinct curvature spheres on each of the linesλ(x,ζ), sinceAζ=0 (see Theorem 2.15).This change in the number of distinct curvature spheres at points of the form (x,ζ) is precisely whyαcannot be extended to a compact proper Dupin submanifold withg=4.

With regard to Theorem 3.2,αcomes as close as possible to satisfying the requirements for being Lie equivalent to an isoparametric hypersurface without actually fulfilling them.The principal curvaturesκ2= 0 andκ4=∞are constant onU.If a third principal curvature were also constant,then the constancy of Ψ would imply that all four principal curvatures were constant, andαwould be the Legendre lift of an isoparametric hypersurface.

Using this same method, it is easy to construct reducible noncompact proper Dupin hypersurfaces inSnwithg= 4 and Ψ =c, for any constant 0＜c ＜1.Ifφ(V) is an isoparametric hypersurface inSn-mwith three distinct principal curvatures, then M¨unzner’s formula (3.2)implies that these principal curvatures must have the values,

Furthermore,any value ofθin(0,π/3)can be realized by some hypersurface in a parallel family of isoparametric hypersurfaces inSn-m.A direct calculation using equations (3.7) and (3.8)shows that the Lie curvature Ψ ofαsatisfies

onU.This can assume any valuecin the interval (0,1) by an appropriate choice ofθin(0,π/3).An open subset of a tube overφ(V) inSnis a proper Dupin hypersurface withg=4 and Ψ=Φ=c.Note that Φ has different values on different hypersurfaces in the parallel family of isoparametric hypersurfaces inSn-m.Thus these hypersurfaces are not M¨obius equivalent to each other by Corollary 2.17.This is consistent with the fact that a parallel transformation is not a M¨obius transformation.

3.4 Local Classifications of Dupin Hypersurfaces

In this section, we mention local classifications of proper Dupin hypersurfaces that have been obtained using the methods of Lie sphere geometry.In the case ofg= 2 principal curvatures, the classification of Dupin surfaces inS3(the cyclides of Dupin) goes back to the nineteenth century.(See, [22, pp151-166] or [9, p148] for more detail.)

A proper Dupin hypersurfaceM ?Snwith two distinct curvature spheres of respective multiplicitiespandqis called a cyclide of Dupin of characteristic(p,q)(see Pinkall[55]).In the same paper, Pinkall [55] proved the following classification of the higher dimensional cyclides(see also [9, pp149-151] for a proof).

Theorem 3.7(a) Every connected cyclide of Dupin of characteristic (p,q) is contained in a unique compact, connected cyclide of Dupin of characteristic (p,q).

(b) Any two cyclides of Dupin of the same characteristic (p,q) are locally Lie equivalent,each being Lie equivalent to an open subset of a standard product of two spheres

wherep+q=n-1.

From this, one can derive a M¨obius geometric classification of the cyclides of Dupin as follows (see [8], [9, pp151-159]).

Theorem 3.8(a) Every connected cyclide of DupinMn-1?Rnof characteristic (p,q)is M¨obius equivalent to an open subset of a hypersurface of revolution obtained by revolving aq-sphereSq ?Rq+1?Rnabout an axisRq ?Rq+1or ap-sphereSp ?Rp+1?Rnabout an axisRp ?Rp+1.

(b) Two hypersurfaces obtained by revolving aq-sphereSq ?Rq+1?Rnabout an axis of revolutionRq ?Rq+1are M¨obius equivalent if and only if they have the same value ofρ=|r|/a, whereris the signed radius of the profile sphereSqanda ＞0 is the distance from the center ofSqto the axis of revolution.

In the caseg=3, Pinkall[52,54](see also the English translation[52]), obtained a classification up to Lie equivalence of proper Dupin hypersurfaces inR4with three distinct principal curvatures of multiplicity one.Another version of Pinkall’s proof in the irreducible case was given later by Cecil and Chern [13] (see also [14], [9, pp168-190]).Cecil and Chern developed the method of moving Lie frames which can be applied to the general study of Legendre submanifolds.This approach has been applied successfully by Niebergall[46,47],Cecil and Jensen[19, 20], and by Cecil, Chi and Jensen [16] to obtain local classifications of higher-dimensional Dupin hypersurfaces.

In particular, Cecil and Jensen [19] proved that for a connected irreducible proper Dupin hypersurface with three principal curvatures, all of the multiplicities must be equal, and the hypersurface must be Lie equivalent to an open subset of an isoparametric hypersurface in a sphere with three principal curvatures.

An open problem is the classification of reducible Dupin hypersurfaces of arbitrary dimension withg= 3 principal curvatures up to Lie equivalence.Pinkall [52, 54], found such a classification in the caseM3?R4,and it may be possible to obtain results in higher dimensions using his approach.

As noted in Section 3.2, local classification in the caseg= 4 has been studied extensively in the papers of Niebergall [46, 47], Cecil and Jensen [19, 20], and by Cecil, Chi and Jensen[16], culminating in Theorem 3.4 of Section 3.2.The method of moving frames is central to the approach of those papers.

As to local classifications in the caseg= 6, an approach similar to that of Cecil, Chi and Jensen [16] is plausible, but the calculations involved would be very complicated, unless some new algebraic insight is found to simplify the situation.

Conflict of InterestThe author declares no conflict of interest.